The Kozeny-Carman equation is one of the most widely accepted and used derivations of permeability as a function of the characteristics of the medium. This equation was originally proposed by Kozeny (1927) and was then modified by Carman (1937, 1956) to become the Kozeny-Carman equation. In essence, this equation will estimate the permeability of media based on a grain size distribution. This equation is given by:
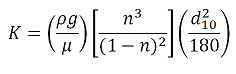
Where
ρ = density
g = acceleration due to gravity
μ = dynamic viscosity
n = porosity
d10 = 10% cumulative passing (geotechnical grain size distribution)
Input
Groundwater temperature estimate: oC
n (porosity):
Click here for information on porosity values
d10 (from a geotechnical plot; % passing): m
Click here for information on d10 and particle sizes
Hydraulic Conductivity: m/s
Example:
A soil matrix has a porosity of 0.30 and a d10 value of 0.1 mm. The groundwater is 10oC. Determine the hydraulic conductivity.
The resulting hydraulic conductivity should be 0.000023 m/s.
What would be a common error in this example?
- forgetting to convert the d10 value from mm to m. The input should be 0.0001 m.